Calculation of Carbon-14 Decay Used in Radioactive Dating

Problem

A sample of wood taken from a freshly felled tree contains 10 grams of carbon. How many atoms of carbon-14 are decaying each second in the sample? The abundance of carbon-14 in atmospheric carbon and in living matter is 1.2 parts per trillion (1.2 x 10^-12) of total carbon. The half-life of carbon-14 is 5,700 years.

Solution

First, let's find the amount of carbon-14 in the sample. There are 10 grams of carbon, most of this is stable carbon-12 and carbon-13. You need to multiply the mass by 1.2 parts per trillion to get the number of grams of carbon-14. To convert grams to a number of atoms, divide by the atomic mass of carbon-14 and multiply by Avagadro's number, 6.02 x 10²³.

number of carbon-14 atoms: 
[10 grams x (1.2 x 10^-12) / (14 grams/mole)] x (6.02 x 10²³ atoms/mole) = 5.2 x 10¹¹ atoms

That's 520 billion atoms of carbon-14.

The half-life is 5,700 years. Let's convert that to seconds and divide by ln(2), the natural logarithm of 2 (0.693), to get the average lifetime of carbon-14 atoms:

average lifetime: 
5,700 years x (365 days/year) x (24 hours/day) x (60 minutes/hour) x (60 seconds/minute) / 0.693 = 2.6 x 10¹¹ seconds

That's 260 billion seconds.

The decay rate (fraction of atoms that decay per second) is the inverse of the average lifetime:

decay rate as a fraction:
1 / (2.6 x 10¹¹ seconds) = 3.8 x 10^-12 per second

The number of atoms decaying per second is the number of carbon-14 atoms multiplied by the decay rate:

decay rate in atoms per second:
(5.2 x 10¹¹ atoms) x (3.8 x 10^-12 per second) = 2.0 atoms per second = 2.0 becquerels

That's 2 atoms per second.


Note: The calculations have a precision of two significant digits. Your answer might be slightly different due to rounding.

Why is carbon-14 decay important?

By measuring the decay rate of carbon-14 atoms in a sample of biological material, you can determine its age. For example, from a sample containing 10 grams of carbon, if you detect 1.0 atoms decayed per second, you can conclude that the concentration of carbon-14 has fallen by one-half, and the sample is one half-life old, or 5,700 years. If the decay rate is 0.5 atoms per second, then two half-lives have elapsed, and the sample is 11,400 years old, and so on.

This method of dating samples is only accurate for up to about 10 half-lives, or 60,000 years. Beyond that, the radioactivity is too hard to distinguish from background radiation and contamination with fresh carbon. For smaller and older samples, a mass spectrometer can be used to count the carbon-12 and carbon-14 atoms individually, rather than relying on detecting the decay of carbon-14 atoms, to get meaningful results.

The formation and decay of carbon-14

Why do only living things have carbon-14, whereas long-dead and non-living carbon samples have no carbon-14? The answer lies in the source of carbon-14. The half-life is 5,700 years, which is very short compared with the age of the Earth, so any carbon-14 existing at the time of the Earth's creation is long gone. The only natural fresh source of carbon-14 is from the action of cosmic rays on atmospheric nitrogen.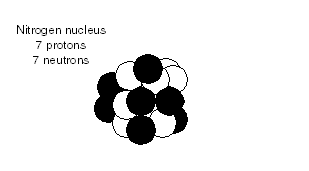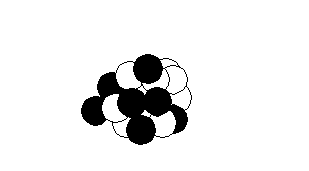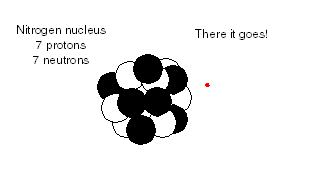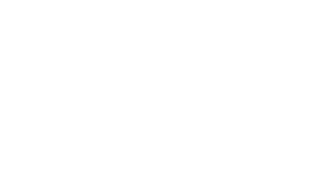

Air is four-fifths nitrogen. The nitrogen nucleus contains 7 protons and 7 neutrons. When a cosmic ray in the form of a neutron strikes an atmospheric nitrogen nucleus, it can knock out and replace a proton.

Then the transformed nucleus has 6 protons and 8 neutrons, which is a carbon-14 nucleus. In other words, the cosmic ray transmutes a nitrogen atom into a carbon-14 atom. This atom separates from its twin nitrogen atom in the N₂ molecule, and then combines with an O₂ molecule to form carbon dioxide.

The newly formed carbon-14 atom is radioactive and has a half-life of 5,700 years (an average life of 8,200 years). When the atom decays, a neutron emits a beta particle (a high-speed electron) and is transformed into a proton. The resulting decay product is a normal nitrogen-14 atom, with 7 protons and 7 neutrons in the nucleus.

Carbon-14 atoms are constantly being created by the action of cosmic rays on atmospheric nitrogen. A the same time, the carbon-14 atoms created by this process are constantly decaying away. Carbon-14 has accumulated in the atmosphere to its equilibrium concentration of 1.2 parts per trillion of total carbon, such that the creation rate and decay rate are the same.

In the 1960s and 1970s, the concentration of carbon-14 in the atmosphere was increased significantly by above-ground nuclear bomb testing. Since such testing has been stopped, the concentration of atmospheric carbon-14 has gradually dropped back to near its natural equilibrium level due to mixing of atmospheric carbon with terrestrial and marine carbon in the carbon cycle.

Uptake of carbon-14 by living things

Plants, by means of photosynthesis, take up carbon dioxide in the atmosphere and use it to make glucose and build up their mass. So all the carbon in plants comes from atmospheric carbon dioxide, and all that carbon contains 1.2 parts per trillion of carbon-14. All animals eat plants, or animals that eat plants, or animals that eat animals that eat plants, and so on. Therefore, carbon-14 travels up the food chain to make all animals as well as plants. As a result, the carbon in all living things contains carbon-14 in a concentration of 1.2 parts per trillion of total carbon.

Decay of carbon-14 in dead things

When a plant or animal dies, it no longer performs photosynthesis or eats, so it  no longer takes up carbon-14 from the atmosphere. There is no replenishment of the carbon-14, even if cosmic rays fall on the dead matter. Remember, it is the action of cosmic rays on nitrogen in the atmosphere that creates carbon-14. Therefore, as soon as the plant or animal dies, the carbon-14 gradually disappears, falling to 0.6 parts per trillion in 5,700 years, to 0.3 parts per trillion in 11,400 years, and so on. 

Meanwhile, the carbon in a lump of coal or a diamond contains no carbon-14 at all. The carbon-14 originally in the dead plant material has decayed away during the millions of years needed for the coal or diamond to form, and no new carbon-14 is being produced there.

Back to Radioactive Decay Problem Examples